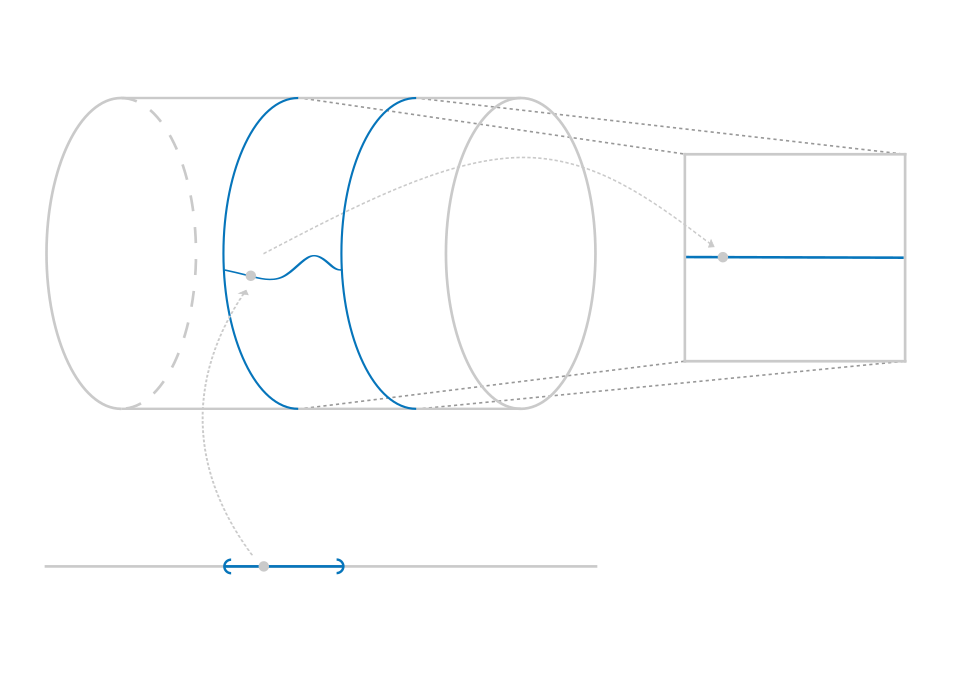
My work is on Yang-Mills theory in the context of symplectic geometry. I leverage recent work on the Hodge theory of primitive forms on symplectic manifolds to study Yang-Mills connections. I am also interested in variants of the Yang-Mills functional and the regularity of their critical points.
My work focuses on the second law of thermodynamics and measures of time-reversal asymmetry through information-theoretic quantities. The centerpiece of my work is an algebraic relationship between information rates and physical observables that quantify the amount of irreversible entropy produced in the time-evolution of a thermodynamic process.
My work focuses on the effects of doping Clifford random quantum circuits with Universal gates. The Universal gates generate the group of all possible quantum logic gates but are costly to implement with current technology. I've constructed a pipeline to manipulate quantum states and circuits for classification by a machine learning algorithm to gain insight on the structure of quantum complexity.